Printable Sudoku
- Alphabet
- Battleships
- Binary
- Bridges / Hashi
- Chain Sudoku
- Consecutive
- Cross Sudoku
- Flower Sudoku
- Frame Sudoku
- Futoshiki
- Galaxies
- Greater Than
- Hitori
- Jigsaw Sudoku
- Kakuro
- KenKen
- Killer Sudoku
- Kropki Sudoku
- Little Killer
- Magic Squares
- Nonogram
- Odd Even Sudoku
- Outside Sudoku
- Rossini Sudoku
- Samurai Sudoku
- Sandwich
- Skyscraper
- Slitherlink
- Sohei Sudoku
- Star Battle
- Sudoku
- Sudoku for Kids
- Sudoku Mine
- Sudoku XV
- Sujiken
- Tripledoku
- Tripod Sudoku
- Twodoku
- Vudoku
- Windmill
Special Variations
Play / Solve
- Home»
- Sohei Sudoku»
- Easy
Easy Sohei Sudoku Puzzles
Collection of free printable easy Sohei Sudoku puzzles to challenge your brain and improve your problem-solving skills. Download and start solving using a pen or pencil and enjoy hours of fun.
- Easy
Showing 1-10 of 10 records
Sort by:
You are browsing category Easy show all
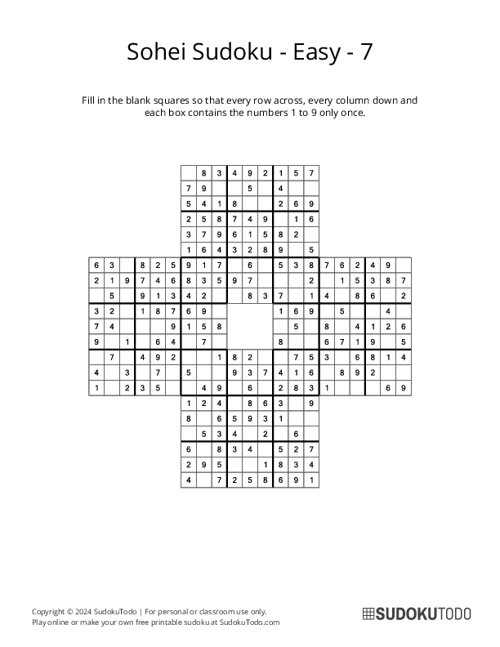
Sohei Sudoku - Easy - 7
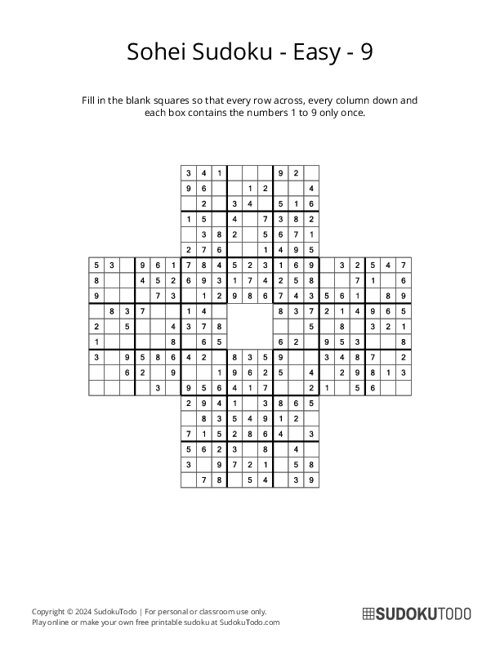
Sohei Sudoku - Easy - 9
Sohei Sudoku - Easy - 4
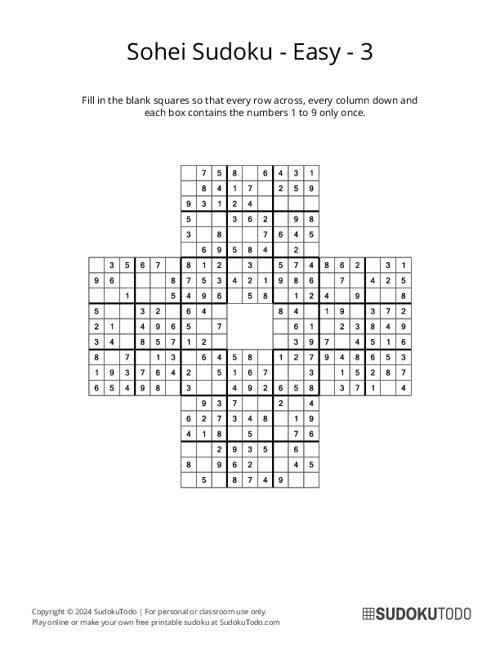
Sohei Sudoku - Easy - 3
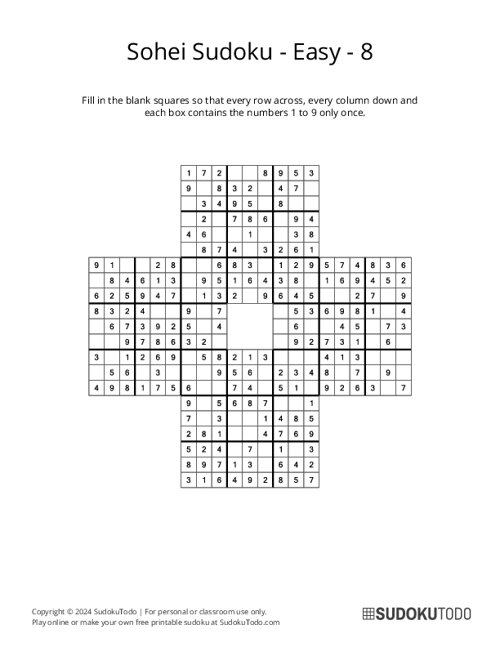
Sohei Sudoku - Easy - 8
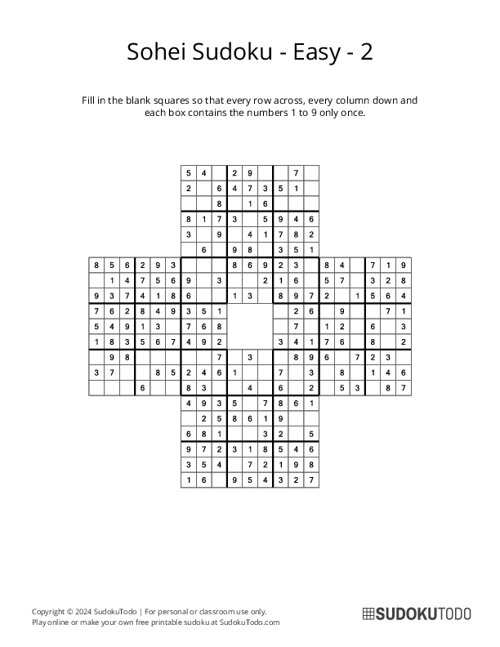
Sohei Sudoku - Easy - 2
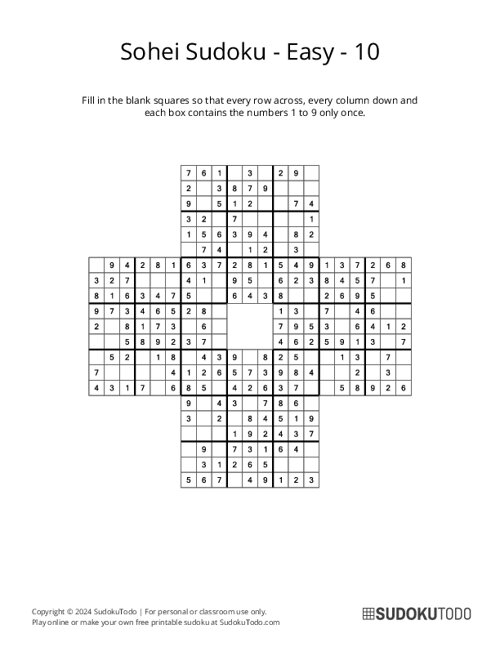
Sohei Sudoku - Easy - 10
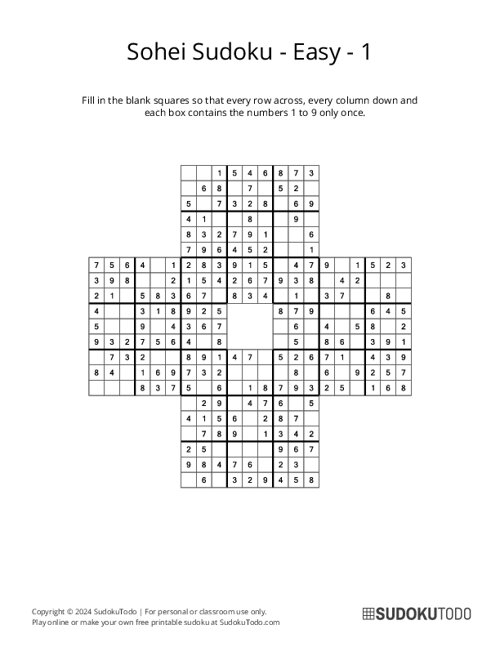
Sohei Sudoku - Easy - 1
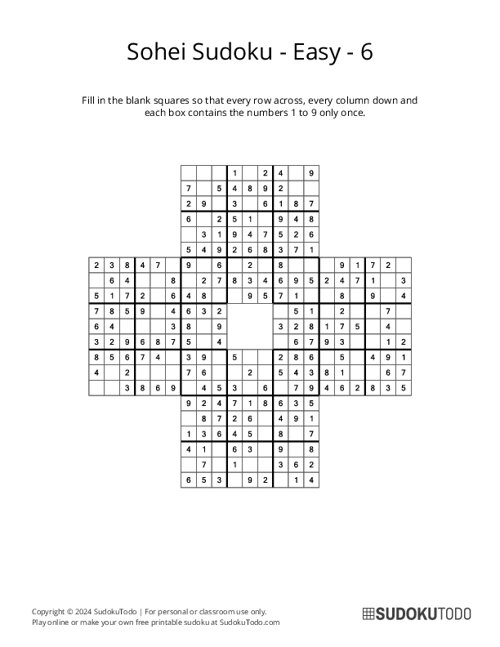
Sohei Sudoku - Easy - 6
Sohei Sudoku - Easy - 5
About Sohei Sudoku
Sohei Sudoku is a special version of the regular Sudoku, named after the warrior monks (Sohei) from feudal Japan. It uses four connected grids instead of just one. These grids overlap in some areas, which means solving one part of the puzzle depends on solving parts of the other grids too.
Besides the usual rules for rows, columns, and 3x3 grids, there are special areas called "sohei" in the puzzle. These sohei regions are groups of cells that also need to be filled with numbers from 1 to 9, just like the rest of the puzzle. However, these regions bring an added challenge.
Basic Rules / How to Play
- Four Interconnected Grids: The puzzle consists of four individual 9x9 Sudoku grids. These grids are strategically merged, creating a larger, interconnected puzzle.
- Unique Numbers: Each row, column, and 3x3 subgrid must contain the digits from 1 to 9 without repetition.
- Interconnected Regions: The grids overlap with each other. The numbers in the overlapping areas must follow the Sudoku rules for both grids.
Solving Tips and Techniques:
To solve Sohei Sudoku puzzles, players use a mix of regular Sudoku methods along with extra strategies to handle the special sohei regions.
- Start with the easier regions: Find sohei regions with fewer blank spaces or ones that are separate (not overlapping with other areas). These are usually the best places to begin.
- Use the edges: The outer cells in sohei regions usually have fewer limitations because they are less likely to overlap with other areas, so it's easier to fill them.
- Naked Single: If a cell in the grid can only contain one number due to the constraints of its row, column, subgrid, and sohei region, fill it in.
- Elimination: If a number has already been placed in a row, column, subgrid, or sohei region, eliminate that possibility for other cells in the same row, column, subgrid, or sohei.
- Pencil Marks: When you can't put a number in a cell, write down all the possible numbers in pencil. Then, as you get more clues, remove the ones that don't fit.
Sohei Sudoku is a fun and challenging version of the classic Sudoku puzzle. It adds special regions called "sohei," which make the puzzles more complex. By using regular Sudoku solving methods and also strategies for the sohei rules, players can solve even the toughest puzzles.