Printable Sudoku
- Alphabet
- Battleships
- Binary
- Bridges / Hashi
- Chain Sudoku
- Consecutive
- Cross Sudoku
- Flower Sudoku
- Frame Sudoku
- Futoshiki
- Galaxies
- Greater Than
- Hitori
- Jigsaw Sudoku
- Kakuro
- KenKen
- Killer Sudoku
- Kropki Sudoku
- Little Killer
- Magic Squares
- Nonogram
- Odd Even Sudoku
- Outside Sudoku
- Rossini Sudoku
- Samurai Sudoku
- Sandwich
- Skyscraper
- Slitherlink
- Sohei Sudoku
- Star Battle
- Sudoku
- Sudoku for Kids
- Sudoku Mine
- Sudoku XV
- Sujiken
- Tripledoku
- Tripod Sudoku
- Twodoku
- Vudoku
- Windmill
Special Variations
Play / Solve
- Home»
- Little Killer»
- Easy
Easy Little Killer Sudoku Puzzles
Download free printable easy little killer sudoku puzzles. Logic puzzles are a fun way to exercise your brain, get better at solving problems, and keep yourself entertained. Perfect for offline play. Download, print and start solving using a pen or pencil.
Showing 1-10 of 10 records
Sort by:
You are browsing category Easy show all
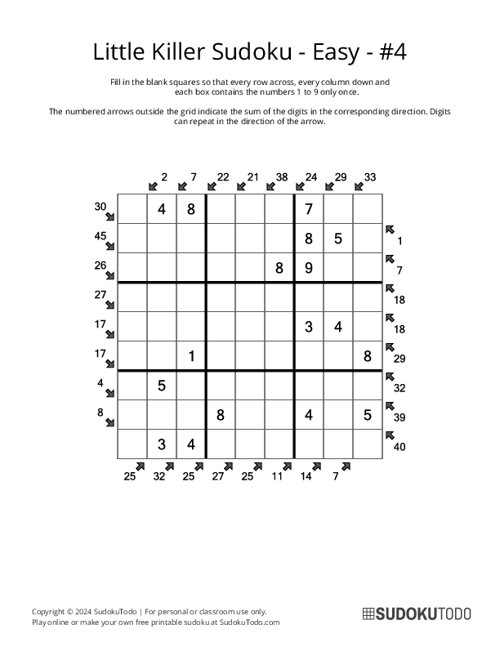
Little Killer Sudoku - Easy - 4
Little Killer Sudoku - Easy - 5
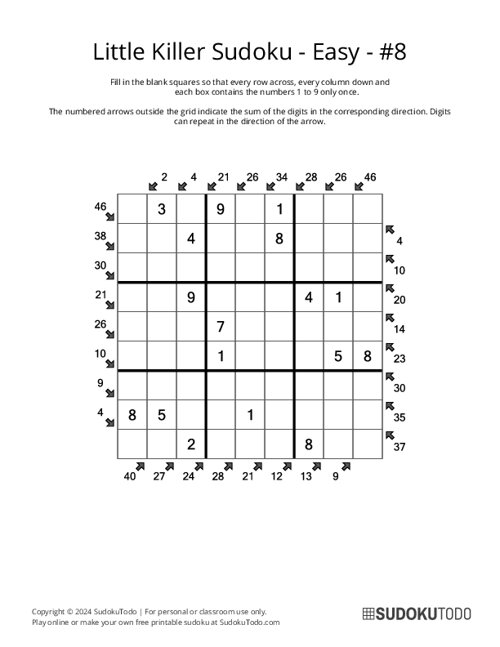
Little Killer Sudoku - Easy - 8
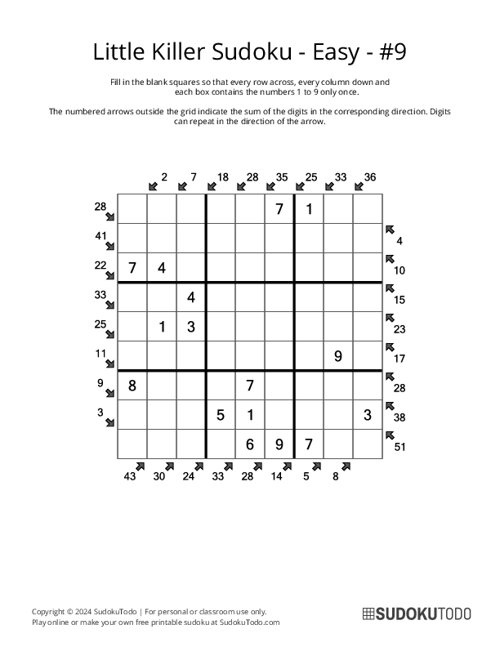
Little Killer Sudoku - Easy - 9
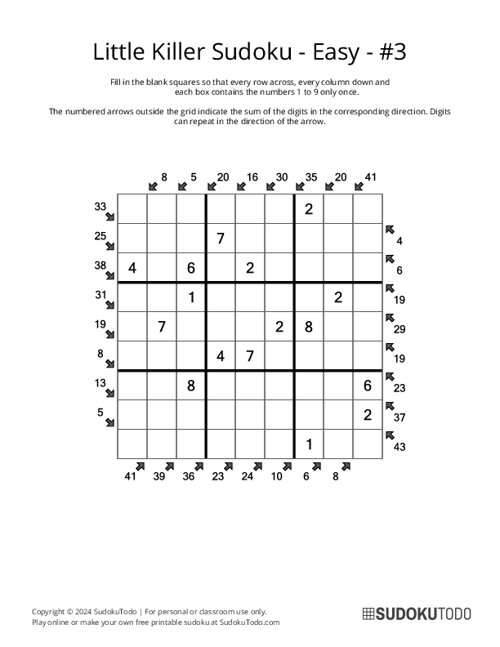
Little Killer Sudoku - Easy - 3
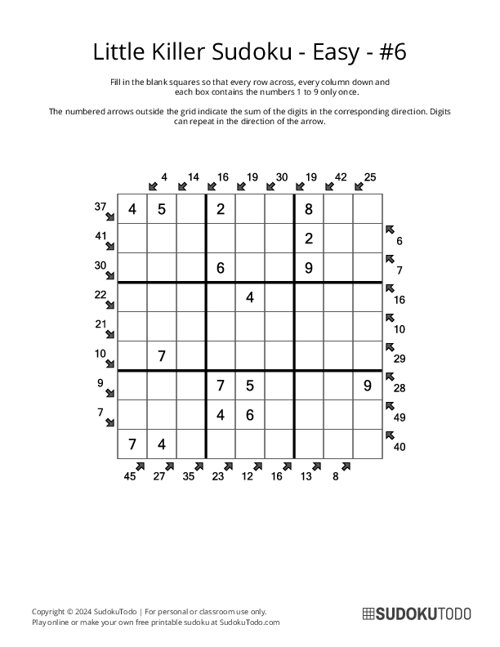
Little Killer Sudoku - Easy - 6
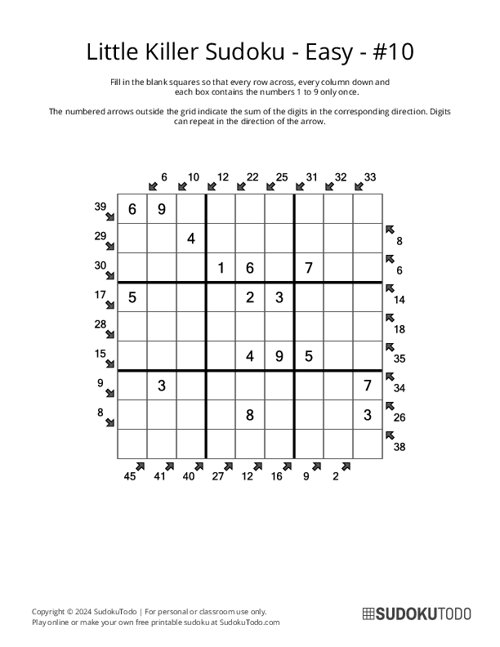
Little Killer Sudoku - Easy - 10
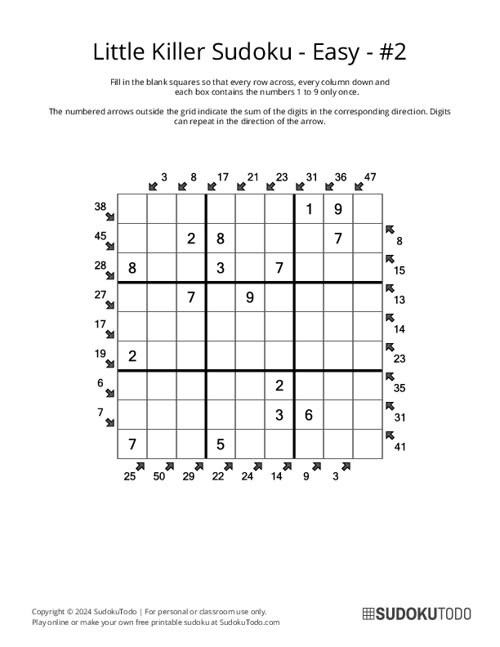
Little Killer Sudoku - Easy - 2
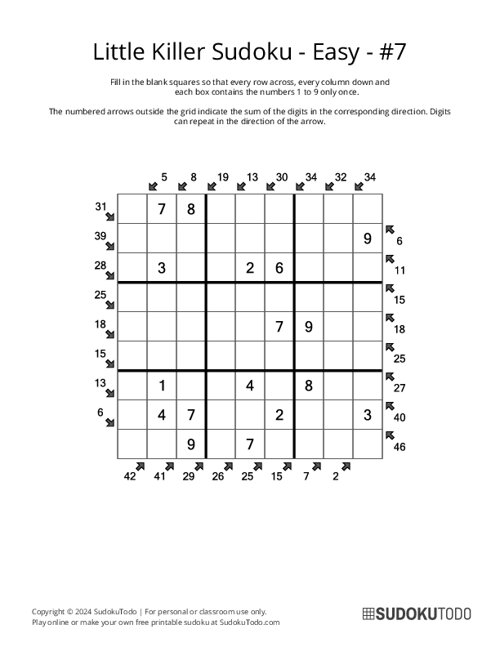
Little Killer Sudoku - Easy - 7
Little Killer Sudoku - Easy - 1
About Little Killer Sudoku
Little Killer Sudoku is a challenging variant of traditional Sudoku. It uses a 9x9 grid, where the numbers 1-9 must appear once in each row, column, and 3x3 box.
Unlike classic Sudoku, this version features arrows outside the grid indicating the sum of digits along diagonals, allowing numbers to repeat (or not repeat in case of unique version of little killer sudoku) along these diagonal paths to match the specified sum.
How to Play
- Grid Size: The puzzle has 9x9 grid size made up of three 3x3 sub-grids.
- Numbers: Each row, column, and 3x3 box must contain the digits 1 through 9.
- No Repeats: Numbers in each row, columns and diagonals should appear only once without repetition.
- Numbers with Arrows: The clues outside of the grid represented by numbers with arrows indicate the direction the sum applies (diagonally across the grid). e.g. If a clue is 10 and the diagonal has two cells, one possible combination is (1,9), (2,8), (3,7), etc.
- Diagonal Numbers: Numbers along a diagonal do not need to be unique, meaning repeats are allowed if they satisfy both the Sudoku and sum constraints.
Solving Tips and Techniques:
- Start with Small Diagonals: Try shorter diagonals first (with fewer cells) as they offer fewer combinations and are easier to analyze.
- Cross Referencing: Use regular Sudoku rules to narrow down options along the diagonals, especially where rows, columns, and 3x3 boxes cross over with a diagonal clue.
- Look for Sum: For each diagonal, look at the given sum to figure out which numbers could add up to it. If the sum is very high or very low, this can narrow down the possible digits that can go on the diagonals.
- Look for Overlapping: Where two or more diagonals overlap, solve for these intersections first to reduce the number of possible values in other cells.